| |
|
 |
 |
 |
| |



Editorial
Meet
the team

Research
networks

Do
Minutes Count for Health Care? Consultation Length in a Tertiary
Care Teaching Hospital and in General Practice
Mothers
knowledge and attitude regarding childhood survival
Is
it a proper referral form?

Diabetes
Mellitus and Angiotensin Converting Enzyme Inhibitors

Human
chorionic gonadotrophin induced Hyperemesis and Hyperthyroidism
in Pregnancy

Family
Medical Centre Patients' Attitudes Toward Senior Medical Students'Participation
in the Examinations
Factors
affecting neonatal death in Fars Province, Southern Iran,
2004
Antibiotics:
Friend Or Foe?

Velocity
and Elasticity Curves of Pregnancy Wastage and Caesarian Deliveries
in Bangladesh
|
| |


Abdulrazak
Abyad
MD, MPH, MBA, AGSF, AFCHSE
Editorial office:
Abyad Medical Center & Middle East Longevity Institute
Azmi Street, Abdo Center,
PO BOX 618
Tripoli, Lebanon
Phone: (961) 6-443684
Fax: (961) 6-443685
Email:
aabyad@cyberia.net.lb
|
| |
 |
| |
Lesley
Pocock
medi+WORLD International
572 Burwood Road,
Hawthorn 3122
AUSTRALIA
Email:
lesleypocock
|
|

 |
 |
|
Velocity
and Elasticity Curves of pregnancy wastage
and Caesarian Deliveries in Bangladesh
|
 |
| |
|
Md. Atikur Rahman
Khan1, Sumaiya Abedin1, Md. Nazrul
Islam Mondal1, and Md. Mostafizur
Rahman2
Institution:
1Department of Population Science
and Human Resource Development, University
of Rajshahi, Rajshahi-6205, Bangladesh.
2Department
of Planning and Statistics, Xiamen
University, Fujian, China.
|
|
ABSTRACT
The aim of this paper
is to investigate the effect of age
of mothers as a cause of pregnancy
wastage and delivery types. Using
the information from 2967 mothers
from Rajshahi District within the
reproductive span (15-49 years), we
have found that the proportion of
pregnancy wastage to live births in
two ages of reproductive years is
tremendously dodgy whereas in other
age groups, between 20 and 35, these
are comparatively benign. Further,
6717 births were studied to investigate
the flow of caesarian deliveries over
the ages. We found 359 caesarian deliveries
against 6,358 natural (vaginal) deliveries.
Some statistical tools were used and
the velocity and elasticity curves
were drawn to analyze the risk of
pregnancy wastage and caesarian deliveries.
Our result shows that the risk of
caesarian delivery increases with
an increased age and this risk expands
with age.
Keywords and phrases:
Pregnancy wastage, Caesarian delivery,
Vaginal delivery, Velocity curve,
Elasticity curve, and Polynomial regression.
|
Pregnancy
is a female state that is produced due to
the implantation of the fertilized ovum
in the uterine endometrium and ultimately
giving rise to a foetus; and pregnancy wastage
is the loss of product of conception normally
or therapeutically (Jeffcoate, 1975). Pregnancy
wastage can be classified as intra-uterine
foetal death, abortion, and menstrual regulation
(Jeffcoate, 1975; and Shaw, Soutter and
Stanton 2003). In our study, we have dealt
with the normal pregnancy wastage that is
not therapeutic.
Every
year about eight million women suffer from
pregnancy related complications and over
half a million die. About 99% of these are
in developing countries (WHO, 2004). Most
of these deaths can be averted even where
resources are limited. The poor reproductive
health of women, in third world countries,
is an outcome of the general neglect of
health and nutrition in childhood and adolescence,
which affects their future well being (De
Silva, 1998). In 1987 Ardebili, Kamali,
Pouranssari and Komarizadeh studied the
reproductive behaviour of 1525 pregnant
women. The type of pregnancy termination
that resulted in live birth or abortion
has a significant relationship to the age
of the mother. Again, the highest percentage
of abortion was observed in (15-19) age
group and the highest number of natural
deliveries was observed in the age group
(20-29).
Khandait
et al (2000) examined complications of the
reproductive health of married women and
envisaged the age factor as a cause of stillbirth,
accumulating with other factors. Yasakawa
and Tayahashi (1990) observed that the age-specific
maternal mortality was rising with age.
They also investigated that pregnant women
aged over 35 faced more pregnancy complications
with high risk. Another study by Breart,
Blondel, and Maillard (1987) acclaimed with
the risks of late pregnancy for mothers
and their births indicated that there was
excess risk for mothers over age 35 but
the difference is decreasing relative to
the general population (mothers before 35).
They also found that the risk of mothers
over 34 was 3.6 times higher in 1975 and
2.6 times higher in 1983 than for the general
population. Study on scheduled caste women
of Punjab experienced the least number of
abortion and stillbirths in the age group
(25-29) and the risk of pregnancy wastage
increases with age (Sidhu and Sidhu, 1988).
Similar results by Banerjee and Hazra (2004)
showed that the rates of pregnancy wastage
in two extreme age groups (<20 and 35+)
are relatively higher. This evidences indicates
that there exists an age-specific relationship
of pregnancy wastage and mothers' age.
Further,
Kim, Byun, and Lee (1991) studied over 2874
mothers and observed 342 caesarian deliveries
against 2532 vaginal deliveries. They included
several factors like, education, occupation,
residence (big cities, urban, and rural)
and age of mother at birth to explain the
delivery status. As to the mothers age at
delivery they found that 10.4% of C-section
(caesarian sections) were under age 24,
12.1% were in (25-29), and 14.1% were over
30. Thus, there is an increasing trend of
C-section with age. But, the exact relationship
of pregnancy wastage and caesarian deliveries
with respect to their flows over the age
of the mother, is still unknown. In this
paper, an attempt has been made to investigate
the age-specific flow of pregnancy wastage
and caesarian delivery.
Data
The data were collected from a field survey
conducted in the district of Rajshahi of
Bangladesh. We collected information from
2967 mothers by preparing an open-ended
questionnaire. Also, the delivery status
of 6717 births were under investigation
and our data evaluated only the delivery
types (caesarian and vaginal).
Methods
In this paper we have used the logic of
equilibrium level of satisfaction, velocity
and elasticity curves. The logic of equilibrium
has been extensively using in the field
of economics especially in demand analyses.
The concept of velocity has a greater applicability
to the physical sciences and recently it
has been used in human biology. On the other
hand, elasticity of goods, demand and income
elasticity, explain the speed of relative
change and replacement (Varian, 2003; Chakravarty,
1997 and Dewett and Chand, 1999).
Equilibrium level
of satisfaction
Equilibrium level of satisfaction leads
to the intersection of two curves at a particular
point. When one curve is downward sloping
and the other is going upward from the origin,
then both the curves intersect each other
at a particular point. At that point values
on both the curves are equal. For example,
if we consider a demand and a supply curve.
Then the equilibrium level of demand and
supply attains at the point of their intersection
(Varian, 2003, Chakravarty, 1997 and Dewett
and Chand, 1999).
Velocity curve
To draw the velocity and elasticity curves
we fit the polynomial regression models.
The velocity curve is just the first derivative
of the fitted polynomial regression line
with respect to age (Gasser et al., 1984;
Ali and Ohtsuki, 2001; Islam et al., 2003).
The polynomial regression model of order
'p' (Gujarati, 1995 and Montegomery and
Peck, 1982) is of the form:

where all the parameters
( ) and the error terms (u) follow the usual
assumptions.
Now, the velocity curve is just the first
derivative of the above equation and so
we get
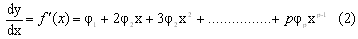
|
|
Elasticity curve
The elasticity can be computed using the
formula mentioned by Dewett and Chand (1999),
Chakravarty (1997) and Varian (2003) as
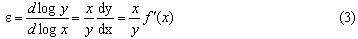
We can comment on the
speed of relative change or replacement
using the following criterion:
| (a) |
if  then the overall process is inelastic,
then the overall process is inelastic, |
| (b) |
if  then the process is elastic and the
speed of relative change is negative,
that is, speed of change of y is slower
than x,
then the process is elastic and the
speed of relative change is negative,
that is, speed of change of y is slower
than x, |
| (c) |
if  then the process is elastic and the
speed of relative change is positive,
that is, the values of y increases faster
than x,
then the process is elastic and the
speed of relative change is positive,
that is, the values of y increases faster
than x, |
| (d) |
if  then the relative change is proportionate
to each other and in this case both
the factors change equally likely.
then the relative change is proportionate
to each other and in this case both
the factors change equally likely. |
Cross validity predictive
power
The cross validity predictive power has
been used to examine the rigidity of the
fitted polynomial regression models. The
cross validity predictive power due to Stevens
(1996) and Khan and Ali (2003) is
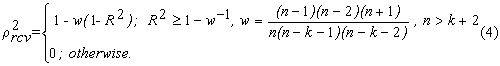
where R2 is
the coefficient of multiple determination,
n is the sample size, k is the number of
regressors used in the model. Further, the
stability of R2(Stevens, 1996,
Khan and Ali, 2003, Islam et al., 2003)
can be computed as: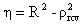
First
of all, we simply present the observed values,
computed proportions (Table 1) and smoothed
proportions (Table 2) both for urban and
rural areas. The proportion of pregnancy
wastage to live birth is calculated by dividing
the number of pregnancy wastage by the number
of live birth in a specific age group. Similarly,
proportion of pregnancy wastage to mother
and that of caesarian deliveries to vaginal
deliveries (Table
1) have been computed. Our aim is
to know the age-specific flow of pregnancy
wastage and caesarian deliveries. So, we
have used smoothing techniques, "4253H,
twice" from Minitab window 12.0 to
obtain the smoothed values (Table
2).
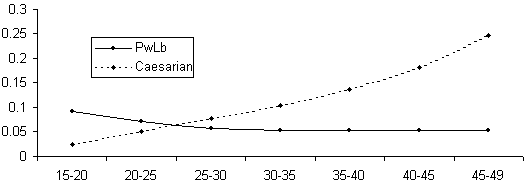
Plotted smoothed proportions
(Fig.1)
intersect each other at a certain point
and at this point equilibrium status is
achieved. We observe that for rural areas,
pregnancy wastage to live birth and to mother,
is equal at age 23. But, in urban areas
this is at age 25. At these points proportion
of pregnancy wastage to live birth and to
mother are equal, that is, number of live
birth equals number of mother. In other
words, at that point every mother yields
a live birth with minor risk of pregnancy
wastage.
A matter of regret is that for delivery
status much data for different age groups
especially the last two age groups, are
not available for urban and rural areas
separately. Thus, we were compelled to conduct
our study combining urban and rural areas
together.Consequently, we have nested our
focus on equilibrium level for proportion
of pregnancy wastage to live birth and proportion
of caesarian deliveries to vaginal deliveries.
At age 25 this equilibrium level is achieved.
Thus, at 25 years of mother  ,
that is, for non-zero pregnancy wastage
(Pw), Live birth (Lb), and Vaginal delivery
(Vd) we have caesarian delivery ,
that is, for non-zero pregnancy wastage
(Pw), Live birth (Lb), and Vaginal delivery
(Vd) we have caesarian delivery  that explains the minor risk of pregnancy
wastage and live birth reduces the risk
of caesarian deliveries. Elaborately, we
can say that live birth and vaginal deliveries
are fixed at certain non-zero level then
increase (or minor risk) of pregnancy wastage
yield increases (or minor risk) of caesarian
delivery. Similarly, if pregnancy wastage
and vaginal delivery is fixed at certain
non-zero levels then the increase of live
birth substantially reduces the risk of
caesarian delivery. Moreover, we can say
that if a woman gives a live birth vaginally
then the risk of caesarian delivery for
the next birth is very low. Here it is mentionable
that these comments are valid only if such
equilibrium condition is achieved. Thus,
our findings elucidate that the women at
age 25 in Bangladesh bears low risk for
child bearing with respect to less risk
of both pregnancy wastage and caesarian
deliveries. To check the liability of our
results we formed a control group that includes
all the respondents of age 25 years. Then
we performed a study over every single year.
One caveat that we faced was availability
of data in every single year; especially
the information on birth and delivery types
for respondents after 35 years of age was
really shaky. However, we did it for those
single year's respondents to whom data were
available. To accumulate the joint effect
of both factors (pregnancy wastage and caesarian
delivery) we added the proportion of pregnancy
wastage to live birth and proportion of
caesarian delivery to vaginal delivery for
every single year. We found that this was
the lowest at the single age year 25 and
was 0.0524. Thus, we may assure that our
results related to the equilibrium level
of age (25 year) are true.
that explains the minor risk of pregnancy
wastage and live birth reduces the risk
of caesarian deliveries. Elaborately, we
can say that live birth and vaginal deliveries
are fixed at certain non-zero level then
increase (or minor risk) of pregnancy wastage
yield increases (or minor risk) of caesarian
delivery. Similarly, if pregnancy wastage
and vaginal delivery is fixed at certain
non-zero levels then the increase of live
birth substantially reduces the risk of
caesarian delivery. Moreover, we can say
that if a woman gives a live birth vaginally
then the risk of caesarian delivery for
the next birth is very low. Here it is mentionable
that these comments are valid only if such
equilibrium condition is achieved. Thus,
our findings elucidate that the women at
age 25 in Bangladesh bears low risk for
child bearing with respect to less risk
of both pregnancy wastage and caesarian
deliveries. To check the liability of our
results we formed a control group that includes
all the respondents of age 25 years. Then
we performed a study over every single year.
One caveat that we faced was availability
of data in every single year; especially
the information on birth and delivery types
for respondents after 35 years of age was
really shaky. However, we did it for those
single year's respondents to whom data were
available. To accumulate the joint effect
of both factors (pregnancy wastage and caesarian
delivery) we added the proportion of pregnancy
wastage to live birth and proportion of
caesarian delivery to vaginal delivery for
every single year. We found that this was
the lowest at the single age year 25 and
was 0.0524. Thus, we may assure that our
results related to the equilibrium level
of age (25 year) are true.
Thereafter,
we fitted statistical models to those smoothed
proportions and found that third order polynomial
regression model better explains the age-specific
flow of both pregnancy wastage and caesarian
deliveries. Our fitted models are highly
stable (Table
3). Further, the velocity and elasticity
of caesarian deliveries and pregnancy wastage
(Table
4) have been computed.
Velocity curves in (Fig.
3) show that velocity of pregnancy
wastage to live birth both in urban and
rural areas yield negative magnitude but
that of caesarian deliveries yield positive
magnitude. Thus, we can say that the pregnancy
wastage decreases over ages whereas caesarian
delivery increases. Elasticity of pregnancy
wastage to live birth is less than unity
up to the age 30 and thereafter these values
lie between -1 and +1, that is, up to age
30 the system is elastic and inelastic thereafter
(Table
4). In other words, the risk of
pregnancy wastage decreases with the increase
of age and the speed is faster than the
speed of age. However, the pregnancy wastage
to live birth in urban areas is almost inelastic
(Fig.4). But, for caesarian deliveries the
elasticity is always greater than one and
so the risk of caesarian deliveries increase
with the increase of age (Fig.4).
Furthermore, the risk of caesarian delivery
(speed) increases faster than the increase
of age.
Risk of pregnancy wastage
and caesarian deliveries change with age.
Increased age increases the risk of caesarian
delivery, but decreases the risk of pregnancy
wastage. However, in the extreme age groups
pregnancy wastages are observed substantially
larger. Equilibrium condition for risk of
pregnancy wastage and caesarian delivery
yields 25 year as an ideal age of child
bearing for Bangladeshi women, as both the
risks are in tolerable situations. Therefore,
no pregnancy before 25 and only one birth
at this age can avail of acceptable risks
of pregnancy wastage and caesarian delivery
in Bangladesh. Different country and regional
differences may draw different risks and
age structure for pregnancy wastage and
caesarian deliveries. Early marriage (before
18 years) and teenage motherhood is a stark
reality behind the plight of female health
hazards in Bangladesh. A proper policy towards
safe motherhood (no birth before 25 years)
may be helpful to overcome the pregnancy
related deficiencies as well as to control
the population growth to a large extent.
A part of this research
has been conducted under a project financed
by the UNFPA. The authors thankfully acknowledge
the Project Director, Dr. J. A. M. S. Rahman
for partial support of our research work.
We are also thankful to the participants
of our seminar for their valuable suggestions.
Last but not least, our sincere gratitude
to the comments from anonymous reviewers
for increasing the rigidity of our paper.
|
 |
| Fig.
1: Smoothed proportion of pregnancy
wastage to live birth and to mother |

Here PwLbU and PwLbR
represent pregnancy wastage to live birth
for urban and rural areas; PwMU and PwMR
refer to pregnancy wastage to mother both
for urban and rural areas, respectively.
<<
back to text
| Fig.
2: Equilibrium level of pregnancy wastage
and caesarian deliveries |
Here PwLb and Caesarian
represent proportion of pregnancy wastage
to live birth and that of caesarian deliveries
to vaginal deliveries, respectively.
<<
back to text
| Fig.
3: Velocity of pregnancy wastage and
caesarian deliveries |
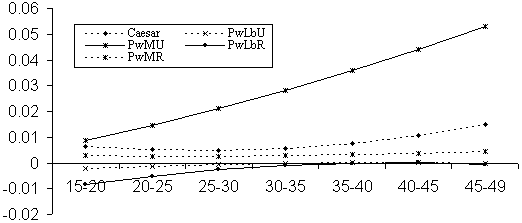
Here Caesar indicates
caesarian deliveries. All other notations
are same as in Fig.1.
<<
back to text
| Fig.4:
Elasticity of pregnancy wastage and
caesarian deliveries |

Here RLB, and ULB indicate
elasticity of pregnancy wastage to live
birth in rural and urban areas,
and C stands for elasticity of caesarian
deliveries in total (urban and rural) areas.
<<
back to text
| Table
1: Pregnancy wastage to mother and live
birth |
|
|
Urban
|
Rural
|
Urban & rural
|
|
Observed Values
|
Observed Values
|
Observed Values
|
|
Age
|
Birth
|
Pw
|
M
|
PwLb
|
PwM
|
Birth
|
Pw
|
M
|
PwLb
|
PwM
|
V
|
C
|
Cv
|
|
15-20
|
21
|
2
|
45
|
0.095238
|
0.044444
|
72
|
8
|
149
|
0.111111
|
0.053691
|
2062
|
51
|
0.024733
|
|
20-25
|
203
|
12
|
202
|
0.059113
|
0.059406
|
341
|
22
|
319
|
0.064516
|
0.068966
|
2297
|
132
|
0.057466
|
|
25-30
|
388
|
23
|
324
|
0.059278
|
0.070988
|
601
|
28
|
351
|
0.046589
|
0.079772
|
1296
|
94
|
0.072531
|
|
30-35
|
523
|
32
|
324
|
0.061185
|
0.098765
|
566
|
30
|
261
|
0.053004
|
0.114943
|
481
|
49
|
0.101871
|
|
35-40
|
519
|
34
|
277
|
0.065511
|
0.122744
|
598
|
21
|
229
|
0.035117
|
0.091703
|
164
|
24
|
0.146341
|
|
40-45
|
446
|
29
|
191
|
0.065022
|
0.151832
|
404
|
17
|
141
|
0.042079
|
0.120567
|
54
|
8
|
0.148148
|
|
45-49
|
268
|
17
|
107
|
0.063433
|
0.158879
|
170
|
7
|
47
|
0.041176
|
0.148936
|
4
|
1
|
0.25
|
|
Total
|
2368
|
149
|
1470
|
|
|
2752
|
133
|
1497
|
|
|
6358
|
359
|
|
Here Pw, M, PwLb, PwM,
V, C, and Cv indicate Pregnancy wastage,
Mother, Proportion of pregnancy wastage
to live birth, Proportion of pregnancy wastage
to mother, Vaginal delivery, Caesarian delivery,
and Proportion of caesarian delivery to
vaginal delivery, respectively.
<<
back to text
| Table
2: Smoothed proportion |
|
AGE
|
Urban
|
Rural
|
Urban & rural
|
Urban & rural
|
|
|
PwLb
|
PwM
|
PwLb
|
PwM
|
PwLb
|
Caesarian to vaginal
|
|
15-20
|
0.078165
|
0.044444
|
0.105083
|
0.053691
|
0.091624
|
0.024733
|
|
20-25
|
0.069348
|
0.059143
|
0.071963
|
0.067360
|
0.070656
|
0.051604
|
|
25-30
|
0.064310
|
0.076591
|
0.051199
|
0.080151
|
0.057755
|
0.077014
|
|
30-35
|
0.063146
|
0.098643
|
0.042820
|
0.093503
|
0.052983
|
0.10428
|
|
35-40
|
0.063337
|
0.123023
|
0.041145
|
0.108585
|
0.052241
|
0.135726
|
|
40-45
|
0.063433
|
0.143879
|
0.041347
|
0.126115
|
0.05239
|
0.180715
|
|
45-49
|
0.063433
|
0.158879
|
0.041176
|
0.147056
|
0.052304
|
0.246312
|
Notations are explained in
Table 1.
<<
back to text
|
Variables |
Fitted Models |
R2 |
CVPP |
Stability |
|
Urban |
Pregnancy wastage to live birthPregnancy wastage to mother |

|
0.99980.9997 |
0.99880.9983 |
0.00100.0014 |
|
Rural |
Pregnancy wastage to live birthPregnancy wastage to mother |

|
0.99970.9999 |
0.99830.9994 |
0.00140.0005 |
|
Both |
Caesarian
deliveries to live birth |

|
0.9998 |
0.9988 |
0.0010 |
<<
back to text
| Table
4: Velocity and elasticity of pregnancy
wastage and caesarian delivery |
|
Age
|
Velocity
|
Elasticity
|
|
PwLb
|
Cv
|
PwLb
|
Cv
|
|
Rural
|
Urban
|
Urban & Rural
|
Rural
|
Urban
|
Urban
and Rural
|
|
15-20
|
-0.00851
|
-0.002327
|
0.006463
|
-1.4141
|
-0.52079
|
4.665101
|
|
20-25
|
-0.00522
|
-0.001331
|
0.005073
|
-1.64836
|
-0.43254
|
2.165553
|
|
25-30
|
-0.00271
|
-0.000596
|
0.004808
|
-1.44058
|
-0.25358
|
1.715809
|
|
30-35
|
-0.00098
|
-0.000122
|
0.005668
|
-0.74046
|
-0.06274
|
1.789762
|
|
35-40
|
-1.57E-05
|
9.14E-05
|
0.007653
|
-0.01449
|
0.054272
|
2.111322
|
|
40-45
|
0.000172
|
4.34E-05
|
0.010763
|
0.176498
|
0.028944
|
2.517524
|
|
45-49
|
-0.00041
|
-0.000266
|
0.014998
|
-0.47722
|
-0.19916
|
2.897614
|
Notations are explained in
Table 1.
<<
back to text
|
|
|
|
| 1. |
Ali MA, Ohtsuki F: Prediction of
Adult Stature for Japanese Population:
A Stepwise Regression Approach. American
J. Hum. Biol., 2001; 13: 316-322.
|
| 2. |
Ardebili HE, Kamali P,
Pouranssari Z, Komarizadeh A: Prenatal
care and maternal age, education and
reproductive behaviour, Iran J Public
Health. 1987;16(1-4):57-64. |
| 3. |
Banerjee B, Hazra S: Socio-demographic
Determinants of Pregnancy Wastage, J
Obstet Gynecol Ind, Vol.54, No. 4: July
/ Aug 2004: 355-360. |
| 4. |
Breart G, Blondel B, Maillard
F: Pregnancy after 34 years: risks and
evolution of risks, Contracept Fertil
Sex (Paris), 1987 Sep;15(9):829-32. |
| 5. |
Chakravarty M: Microeconomics:
Theory and applications, 1997; Vol.1:
349-413. |
| 6. |
De Silva WI: Symptoms
of Ill Health and Health Seeking Behavior
of Sri Lankan Mothers during the Puerperium:
Asia Research Institute, National University
of Singapore, 1998. |
| 7. |
Dewett KK, Chand P: Modern
Economic Theory, 21st revised ed., S.
Chand and Company Ltd., New Delhi, 1999. |
| 8. |
Gasser T, Kohler W, Muller
HG, Kneip A, Largo R, Molinari L, Prader
A: Velocity and accelaration of height
growth using kernel estimation. Ann
Hum Biol, 1984; 11:397-411. |
| 9. |
Gujarati D N: Basic Econometrics,
3 rd ed., McGraw Hill, Singapore, 1995:
217-221. |
| 10. |
Islam MR, Islam MN, Ali
MA, Mostofa MG: Construction of Male
Life Table from Female Widowed Information
of Bangladesh. International Journal
of Statistical Sciences, 2003; 2: 69-82. |
| 11. |
Jeffcoate SN: Principles
of Gynaecology, 4th ed., Butterworths
World Student Reprints, Boston, 1975. |
|
|
| 12. |
Khan MAR, Ali MA: Restricted cross
validity predictive power, Pakistan
Journal of Statistics, 2003; Vol.
19, No. 2:199-202.
|
| 13. |
Khandait DW, Ambadekar
NN, Zodpey SB et al: Maternal age a
risk factor for still birth, Indian
Journal of Public Health, 2000; 44:28-30. |
| 14. |
Kim ES, Byun YC, Lee SH:
A study on socioeconomic factors related
to cesarean section in Korea, Bogeon
sahoe nonjib. 1991 Dec;11(2):19-35. |
| 15. |
Montegomery DC, Peck EA:
Introduction to linear regression analysis,
John Wiley and Sons, New York, 1982. |
| 16. |
Sidhu S, Sidhu LS: Pregnancy
wastage in scheduled caste women of
Punjab, Ann Hum Biol., 1988 Mar-Apr;15(2):167-70. |
| 17. |
Shaw RW, Soutter WP, Stanton
SL: Gynaecology, 3rd ed, Edinburgh :Churchill
Livingstone, 2003. |
| 18. |
Stevens J: Applied multivariate
statistics for social sciences, Lawrence
Erlbaum Associates, Inc., Mahwah, New
Jersey, 1996. |
| 19. |
Varian HR: Intermediate
Microeconomics A modern Approach, WW
Norton and Company, Inc., 2003. |
| 20. |
Yasakawa T, Hayashi K:
Trend analysis of maternal mortality
in Japan in relation to biological factors,
Koshu Eisei In Kenkyu Hokoku. 1990 Mar;39(1):11-9. |
| 21. |
WHO: World Health Organization,
Geneva, 2004. |
|
| |
|
|
|
|